Francesco Maria Grimaldi (continued)
The discovery of diffraction
Shadows projected by opaque bodies illuminated by solar light or by point sources built in the laboratory lead to the conclusion that, in a transparent homogeneous medium, light propagates along straight lines (the law of rectilinear propagation). This conclusion, however, does not hold rigorously and without limits.
Grimaldi was the first to realize
it, in the course of his attempt to subject to careful examination the way
light rays were conceived in those days: they ought to be "straight lines"
indeed, but not quite in the strict sense the term has in geometry, in so far
as they should have a certain width in order to represent something physical.
In order to check this idea, Grimaldi drew a little hole in the shutter of a
window, thus letting the sun's light into a room (here we have almost his own
words). Propagation of light in the room would then take place along a cone
"that shall be visible in the air if there be some dust or if some smoke is
produced". Interposing an opaque body along the light's path will produce a
shadow on a sheet of white paper spread perpendicular to the direction of the
cone's axis. However – and in this consists the first important aspect of the
discovery – "the limit of the shadow remains in some way ill-defined, due
to a certain penumbra, characterized by a perceptible graduation [...] in the
regions between total shadow and full light [...]". Moreover, the overall shadow
appeared consistently wider than it ought to be on the assumption that
"everything took place in terms of straight lines".
|
|
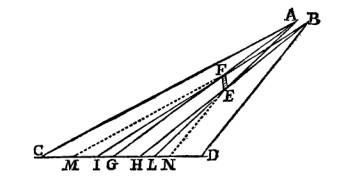
These aspects are discussed by Grimaldi with the help of the diagram on
the left, taken from De lumine.
Referring to the diagram, Grimaldi also pointed out that in the regions CM and ND light appeared to open to some extent fanwise, in the sense that it concentrated (in "fringes") around certain privileged directions; in the middle of each fringe light appeared "pure and genuine", whereas it presented some colour at the edges. Finally, the fringes showed a certain dependence on the width of the hole, and disappeared if it became too large. |
|
| Fig. 1: AB is the hole from which light penetrates into the room: EF is the obstacle across the light cone; MN is the projected shadow, sensibly wider than that foreseen by the law of rectilinear propagation; IG and HL are the zones of penumbra, GH that of full darkness. |
See how, in the passage that follows - taken from a recent textbook of general physics - the reader is introduced to the subject of deviations from the law of rectilinear propagation of light:
<< Let us consider, for instance, the shadow projected from the edge of an opaque object. If the light source is point-like, then, according to this law, one should expect to obtain on the screen a sharp frontier between the dark and the light regions. Actually, there exists a transition area in which the degree of illumination varies in a continuous and non-monotonic way: here "diffraction fringes" are observed>>
We can see that the situation described and the description itself are extremely similar to those given by Grimaldi.
 |
Fig. 2: Figure of diffraction produced by a razor blade illuminated with a blue light from a laser. |
|
|